Programming Languages
in CS 101 on Programming Basics
Let’s look at the tools a computer needs to understand high-level (that means, a little like English) programming languages. Then, let’s discuss language paradigms, how languages can be grouped by their features in various ways.
How Computers “Understand”
We know that computers only speak binary. So, when we write and execute programs in high-level programming languages (such as Python), we need several tools to translate. This is the stages of ‘life’ for a computer program.
- Editors: A program is ‘born’ or begins in a text editor where we write our code. This stage is like writing an essay in MS Word. Instead, we’re writing code that means something (or, after it’s translated in to binary, will mean something) to a computer.
- Compilers: When a program is compiled, it is converted into machine-code. A program written in a high-level language can run on any computer that has an appropriate compiler (aka the program that translates the language into machine code).
- Machine-code is also a computer programming language (but a very low-level language because it doesn’t resemble English very much) consisting of binary or hexadecimal instructions that your computer can respond to directly.
- In the early days of computer programming, the output of a compiler was an assembly-language version of the program, when then had to be passed through to an assembler to finally get to machine-language. Today, compilers are more sophisticated and the assembly-language phase is often eliminated.
- You can read more about compilers and other tools in my post on theLife of a C++ Program
- Interpreters: is a program that translates and executes statements in sequence. In other words, it directs the computer to perform the actions specified in your program.
Programming Language Paradigms
There are many programming languages. Therefore, grouping languages together by common characteristics can help us understand the larger picture. A paradigm is simply a group. There are two main language paradigms, imperative and declarative, and many subparadigms within each.
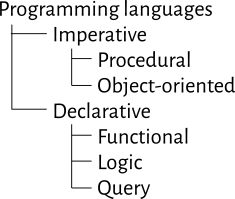
Imperative Languages
The von Neumann model of sequential instructions operating on values in memory greatly influenced the most common model of programming language: the imperative paradigm. Imperative languages work by issuing a sequence of commands/instructions for the computer to understand. In Python, each statement we type is a new command, telling the computer to print some text, do some calculation, or update a variable.
def fact(n): # Factorial function, imperative style, Python
k = 1
while n > 1:
k = k * n
n = n - 1
return k
Procedural Languages
The imperative language family is further divided into procedural and object-oriented languages. In procedural languages, the program is divided into procedures aka groups of steps to solve a particular problem. Procedures are also called subprograms or functions. Our pseudocode examples follow this model.
Object-Oriented Languages
Object-oriented languages see the world as a bunch of interacting objects. Each object is responsible for its own actions and can contain (aka encapsulate) procedures and data structures within themselves. Furthermore, various objects in a program can send messages and respond to each other’s messages in order to solve problems.
Declarative Languages
In the declarative paradigm, results are described (aka declared) and the steps to accomplish those results are not stated. There are two main declarative subparadigms, functional and logic languages.
Functional Languages
The functional model uses math to describe functions. This means that each piece of code throughout a program produces a value and can be replaced with its value without any side effects.
(define factorial ; Factorial function, functional style, Scheme
(lambda(n)
(if
(= n 0)
1
(* n (factorial (- n 1))))))
fact n = product [1..n] -- Factorial, functional style, Haskell
Logic Languages
Logic programming is based on predicate logics, a branch of math that deals with formal reasoning about AND and OR (the Boolean operators), as well as implication, relations, and inference. In other words, this model uses facts about objects and rules about the relationships among the objects. A program would consist of asking questions about these objects and their relationship, where answers are deduced from the facts and rules.
/* Example clauses in Prolog*/
likes(mary,food).
likes(mary,apples).
likes(john,apples).
likes(john,mary).
The following questions (aka queries) give these answers:
| ?- likes(mary,food).
yes.
| ?- likes(john,apples).
yes.
| ?- likes(john,food).
no.
Query Languages
Query languages characterize interaction with a database such as inserting, updating, deleting, selecting records. SQL (Structured Query Language) is a famous query language that we’ll learn about later.
Expression Notation
One way in which some languages differ is in how arithmetic expressions are written. The way we’re familiar with is called infix notation because we put the operator in between the operands. For example, a + b.
However, there are alternative ways of writing arithmetic expressions: prefix and postfix.
In the Lisp language family (which includes Scheme, Racket, and others), we use prefix notation. This means the operator goes before the operands. Instead of writing 1 + 2 * 3, we write (+ 1 (* 2 3)).
You evaluate prefix expressions starting from the innermost parentheses, like this:
(+ 1 (* 2 3)) ⇒
(+ 1 6) ⇒
7
On the other hand, some languages use postfix notation. No parentheses need, just specify the numbers (separated by spaces) and the operators after. Instead of writing 1 + 2 * 3, we write 1 2 3 * +